hatingphysics
- 14
- 0
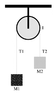
In an Atwood machine, one block has a mass of M1 = 490 g and the other has a mass of M2 = 290 g. The frictionless pulley has a radius of 5.1 cm. When released from rest, the heavier block moves down 65 cm in 1.15 s (no slippage).
What is the tension T1?
Find the pulley's moment of inertia.
I found the magnitude of the acceleration of the lighter block (9.83×10-1 m/s^2 ) and the magnitude of the angular acceleration of the pulley (1.93×101 rad/s^2 ) but why can't I find the other 2 answers...HELP!
What is the tension T1?
Find the pulley's moment of inertia.
I found the magnitude of the acceleration of the lighter block (9.83×10-1 m/s^2 ) and the magnitude of the angular acceleration of the pulley (1.93×101 rad/s^2 ) but why can't I find the other 2 answers...HELP!