member 731016
- Homework Statement
- Please see below
- Relevant Equations
- ##F = mg##
For this problem,
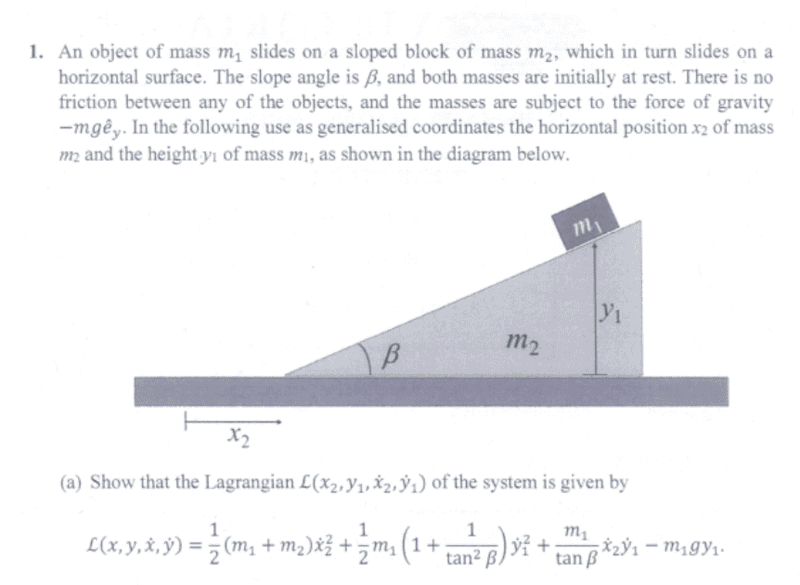
I am confused where they get these two terms from

Can someone please explain? I get all the other terms expect those.
Thanks alot!
I am confused where they get these two terms from
Can someone please explain? I get all the other terms expect those.
Thanks alot!