zenterix
- 774
- 84
- Homework Statement
- I'm having a bit of trouble with calculating two specific limits.
Here is some context about where the limits came from, though my question is strictly about how to calculate the limits.
I was asked to calculate the electric field at a point ##P##, located a distance ##y## from the center of a rod along its perpendicular bisector (see image below).
- Relevant Equations
- The answer is the following expression for the electric field at point ##P##:
$$\vec{E}_p=\frac{k_eQ}{y\sqrt{y^2+\frac{l^2}{4}}}\hat{j}$$
I would like to calculate the limit of this expression in two edge cases:
1) ##y>>l##
2) ##l>>y##
The answers are ##\frac{k_eQ}{y^2}\hat{j}## and ##\frac{2k_e \lambda}{y}\hat{j}##, respectively, where ##\lambda## is simply the linear density of the rod, ie ##\frac{Q}{l}## (again, this is just for context).
I know what the answers are, because this is all part of the notes from MIT OCW's 8.02 Electromagnetism course. In case you want to see the actual problem, it is example 2.3 that starts on page 18; the limits I am asking about are on page 20.
How do I go about calculating the limits? Ie, what is the name of the specific technique from Calculus used to solve them? I think it might be a Taylor expansion of part of the denominator of the expression for ##\vec{E}_p##.
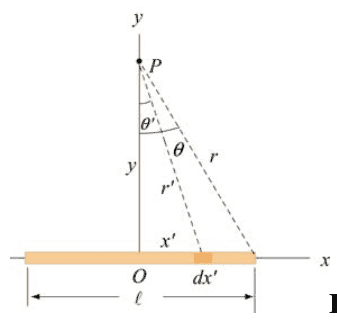
How do I go about calculating the limits? Ie, what is the name of the specific technique from Calculus used to solve them? I think it might be a Taylor expansion of part of the denominator of the expression for ##\vec{E}_p##.