tracker890 Source h
- 90
- 11
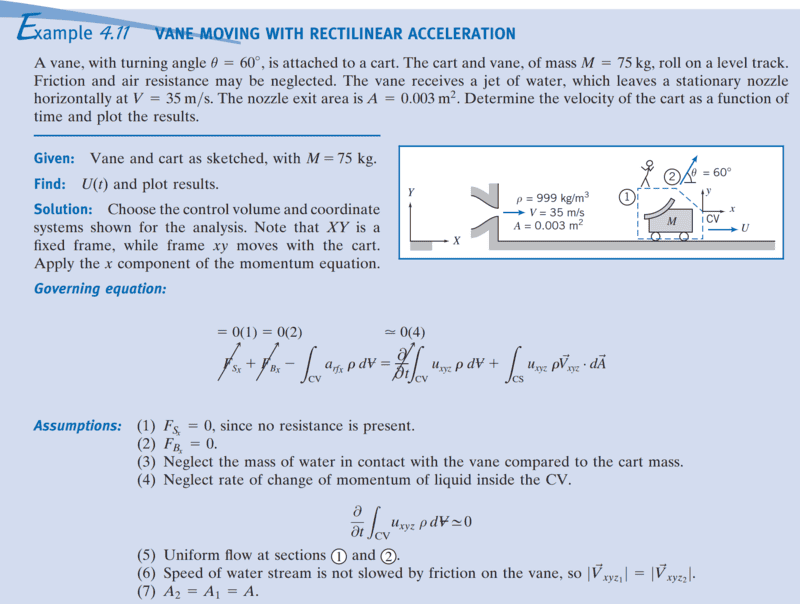
- Homework Statement
- It's not apparent why the fluid velocity in the relative non-inertial reference frame is zero.
- Relevant Equations
- Momentum equation in non-inertial coordinates
Q: Regarding item (4), my understanding aligns with (eq_1), where M is a constant. However, why does ##\left( \frac{\partial}{\partial t}u_{xyz} \right)## in (eq_1) equal 0?
$$
\frac{\partial}{\partial t}\int_{CV}^{}{u_{xyz}}\rho d\forall =\frac{\partial}{\partial t}\left( u_{xyz}\cdot M \right) =M\left( \frac{\partial}{\partial t}u_{xyz} \right) =0\cdots \text{(}eq\_1\text{)}
$$
reference
$$
\frac{\partial}{\partial t}\int_{CV}^{}{u_{xyz}}\rho d\forall =\frac{\partial}{\partial t}\left( u_{xyz}\cdot M \right) =M\left( \frac{\partial}{\partial t}u_{xyz} \right) =0\cdots \text{(}eq\_1\text{)}
$$
reference