Scalar fields/ Scalar functions / Vector fields / Vector functions

[K41]

I know that physically, they describe relationships whereby, for instance a vector field, for each point in three dimensional space (a "vector"), we have a "vector" which has a direction or magnitude.

Now I once asked what the difference between a vector field and a vector function is and the definition was given below:

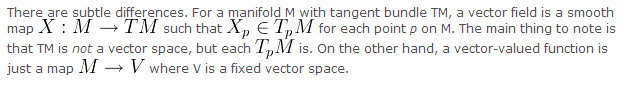
The problem is, I still can't picture it for a simple vector field:

V(x,y) = u(x,y)i + v(x,y)j

Someone later on did explain what the definition meant but I still can't put two and two together.

So what is a tangent bundle and what is a vector (or topological) space in relation to a fluid flow. In terms of physics, it sounds to be like we have a global fixed reference frame which has its own x,y,z co-ordinate system and then we have a local co-ordinate frame which is, for this example, attached to a fluid element which then has a vector associated with it. But then why do we need a manifold and why do we need things to be tangent to it? If indeed the vector field maps from a manifold to some vector space, why do the vector spaces have to be attached to the manifold, because wouldn't that mean we can't place our global reference system anywhere in the flow field? Finally, why is the tangent bundle (presumably the collection of all the tangent or vector spaces) not a vector space in itself? So following on from that, can anyone give the precise definition for scalar fields and scalar functions and then tell me why they are different (mathematically rather than saying it doesn't have a direction etc).

PS: I know there is some 2007 thread in these forums but I didn't really get it and its locked anyway.

 

[Studiot]

OK here we go. a simple man's guide A
You are asking about the difference between vector valued functions (not vector functions, they are different and I will come to that) and scalar functions (not scalar valued functions they are again different).

A function has a domain, a co-domain and a rule.

It is a mapping from an element or member in the domain set to the a unique element of the co-domain set, according to the rule.

Note that whilst many elements in the domain may map to the same element in the co-domain, each element in the domain may only map to one element in the co-domain.

That sounds like a mmouthful - and it is- but the rules are simple.

Now the members of the domain set may be scalars, vectors or something else.

Also the members of the co-domain set may be vectors, scalars or something else.

Now for the clever stuff.

If the domain and co-domain elements are scalars, the function is a scalar function
If the domain and co-domain elements are vectors, the function is a vector function

If the domain elements are scalars and the co-domain elements are vectors the function is a vector valued function.

If the domain elements are vectors and the co-domain elements are scalars the function is a scalar valued function, also called a functional.

To try to get a geometric picture, the position domain using R, R² or R ³ are scalars.

If we assign a scalar to each point this is a simple number and exists in the space Rⁿ⁺¹⁾

If we assign a vector to each point in R³, those vectors cannot exist in the same R³ (domain) as the positions.
This is what is meant by tangent bundles etc.

If you have followed the story so far we can proceed.

 

[K41]

Note that whilst many elements in the domain may map to the same element in the co-domain, each element in the domain may only map to one element in the co-domain.

Does that mean f(x) = 4 is not a function? What is it called then :s

To try to get a geometric picture, the position domain using R, R² or R ³ are scalars.

If we assign a scalar to each point this is a simple number and exists in the space Rⁿ⁺¹⁾

If we assign a vector to each point in R³, those vectors cannot exist in the same R³ (domain) as the positions.
This is what is meant by tangent bundles etc.

If you have followed the story so far we can proceed.

I don't get that. So we call the domain a position domain. What does that mean? Also, okay so we have some elements in this domain, why can't we have vectors at these points as well? Like a vector has a co-ordinate in a reference system and then it has a direction from that point of a certain magnitude as well??

 

[Studiot]

f(x) = 4 is a perfectly respectable function that may be admitted to the best function clubs.

It says that every member of the domain is mapped to the number 4 in the co-domain.

Your response suggests you are not familiar with the definition and terminology of functions.
Would you like to review them?

I didn't say you can't associate a vector with a position in space.
I said that if you do the vector can't live in the same space as the point, with the exception of the displacement vector and the position vector. All other vectors live in a different space.

Consider the point (1, 1)
Now associate a vector of length √2 at that point.

where is the other end of the vector? at the point (2,2) or (0,0) or where?

Look at post#13 of this thread

https://www.physicsforums.com/showthread.php?t=640080&highlight=vector

 

[K41]

f(x) = 4 is a perfectly respectable function that may be admitted to the best function clubs.

It says that every member of the domain is mapped to the number 4 in the co-domain.

Your response suggests you are not familiar with the definition and terminology of functions.
Would you like to review them?

Yes, if its not too much trouble thanks. I thought the above functions were were dealing with were meant to be one to one only.

I didn't say you can't associate a vector with a position in space.
I said that if you do the vector can't live in the same space as the point, with the exception of the displacement vector and the position vector. All other vectors live in a different space.

Consider the point (1, 1)
Now associate a vector of length √2 at that point.

where is the other end of the vector? at the point (2,2) or (0,0) or where?

Look at post#13 of this thread

https://www.physicsforums.com/showthread.php?t=640080&highlight=vector

I wasn't quite sure about post 13. I still don't get why the force components aren't in the XY plane? Why are they different to the geometric vectors D1 and D2? Why would vectors represent areas anyway, wouldn't they represent volumes?

I think I get what you mean by vector space and how if you only specify the magnitude from one point, the end point of the vector can have two different points. Is that why its different from "position" space - because we have two different values and no unique value?

Are the position space and vector space still in Euclidean space?