- 4,662
- 372
- TL;DR Summary
- My question is referring to some derivation from pages 293-294 of Schutz's second edition (2009) of A First Course in GR.
In the screenshots below there are the equations (11.49) and (11.53).
I don't understand how did he derive equation (11.53) from Eq.(11.49)?
From (11.49) I get: ##d\phi/dy= d\phi/du du/dy = (1/b^2-u^2+2Mu^3)^{-1/2}(1+2My)##.
It seems he neglected the ##2Mu^3## since ##Mu\ll 1##, so ##y\approx u##, but how do we get the added term of ##O(M^2u^2)##?



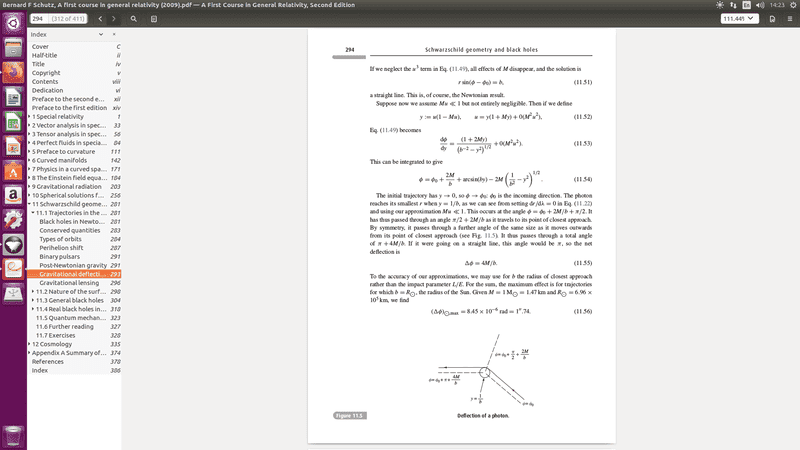
I don't understand how did he derive equation (11.53) from Eq.(11.49)?
From (11.49) I get: ##d\phi/dy= d\phi/du du/dy = (1/b^2-u^2+2Mu^3)^{-1/2}(1+2My)##.
It seems he neglected the ##2Mu^3## since ##Mu\ll 1##, so ##y\approx u##, but how do we get the added term of ##O(M^2u^2)##?

