- #71
sysprog
- 2,617
- 1,796
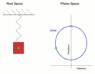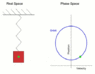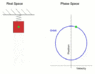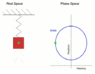
That's a definitional difference. The mass rotates about point ##\text A## in either case. Removing the gravity would make the rotation about point ##\text A## circular with the center at point ##\text A##, instead of the rotation being circular about the point formerly called point ##\text C##. In discussing the effect of removing gravity, I regarded point ##\text C## as a static point already defined as in the original problem. You're using a dynamic definition of point ##\text C## to mean the center of the circle of rotation, wherever that is. The effect of removing gravity is the same by either description.haruspex said:No, in the absence of gravity A and C would be the same point.