- #1
MatinSAR
- 632
- 190
- Homework Statement
- How can I find work done by variable force using F.dr When the equation of path is x=(2a) sinθ and y=(a) cosθ and F=(x^2) y i + x(y^2) j? If you can guide me how to write the integral relation so that I can calculate it, I would be grateful.
- Relevant Equations
- W=Int(F.dr)
Can anyone tell me if my answer is wrong ?!
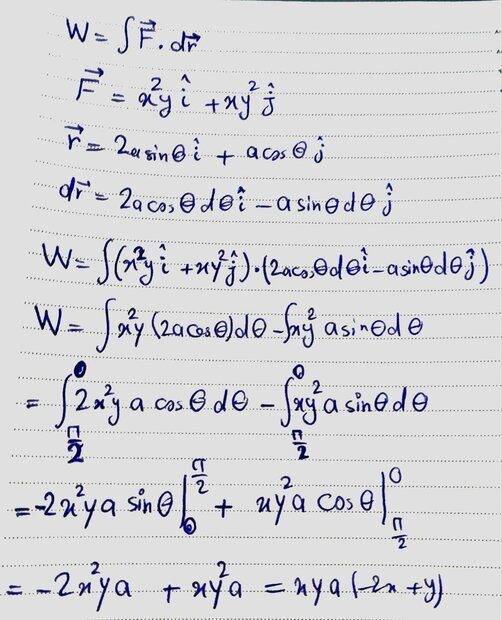
Last edited: