You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Angular Definition and 999 Threads
-
K
I How Does the Book's Formula for Angular Momentum Differ from Mine?
A disc initially has angular velocities as shown It's angular momentum along the y-axis initially is ##L_s## I tried to find its angular momentum and ended up with this:##L=I_{x} \omega_{x}+I_{y} w_{y}+I_{z} z_{z}##The z component of angular momentum is thus ##L_{z}=I_{z} \omega_{z}## However... -

I Apparent angular velocity (inclined orbit)?
Here's the problem setup, my student and I are stuck. A disk is rotating at constant angular velocity ω, and we are watching a point on the rim, parameterized by the angular position θ, move. Because we are observing the motion from an inclination angle Ψ, we do not always observe the...- Andy Resnick
- Thread
- Replies: 11
- Forum: General Math
-
F
A Relation between Matter Power spectrum and Angular power spectrum
From a previous post about the Relationship between the angular and 3D power spectra , I have got a demonstration making the link between the Angular power spectrum ##C_{\ell}## and the 3D Matter power spectrum ##P(k)## : 1) For example, I have the following demonstration, ## C_{\ell}\left(z... -
B
Existential dilemma on angular velocity of a complex rigid body
I assumed the angular velocity of the center of mass of the two discs about z axis to be w1 note that angular velocity of center of mass of both discs and center of anyone disc about z axis is same, you can verify that if you want, me after verifying it will use it to decrease the length of the...- bhupesh
- Thread
- Replies: 25
- Forum: Introductory Physics Homework Help
-
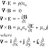
Measure angular velocity and acceleration from missing tooth wheel
Hi, I need to come up with a math model for a digital ignition system. I've been thinking about it and I think that I need to measure 2 things to be able to calculate when I have to start charging the coil. They are the angular velocity and the acceleration but how can I do it? the idea is to... -

B Electron angular momentum, gyroscope?
Any spinning item, proton, electron, even planet, has angular momentum that creates force. How can an electron exist in a random orbital cloud around a spinning proton if it has an angular momentum and requires force to alter from any circular orbital plane (like a planet orbiting a star)?- Homestar1
- Thread
- Replies: 14
- Forum: Quantum Physics
-

Analyzing an Angular Impulse Problem
What we know: The ball is dropped at the tip A with some speed ##v_0## and rebounds with speed ##v##. This collision produces an angular impulse, changing the angular momentum of the bar with the flywheels. Solution inspired by an answer provided by @TSny in the similar question. Angular...- PiEpsilon
- Thread
- Replies: 12
- Forum: Introductory Physics Homework Help
-

Terminal angular velocity of Disc in magnetic field
Torque appiled by smaller disc = mga emf of disc due to B = Bwr2/2 Current I = Bwr2/2R force = IBr = Bwr3/2r torque = rF = Bwr4/2r mga = Bwr4/2r- Advay
- Thread
- Replies: 1
- Forum: Introductory Physics Homework Help
-
V
How to determine angular velocity about a certain axis?
If the crawling insect were stationary at a certain instant of time, then it would have the same angular velocity as that of disk, which is w in a clockwise direction. But now it's velocity at any instant is the vector sum of velocity due to rotation and the velocity it crawls at. My attempt is...- vcsharp2003
- Thread
- Replies: 10
- Forum: Introductory Physics Homework Help
-
H
Angular Frequency of a two-object system
I calculate as follow and get a correct answer, but I wonder why the weight of the ladder 6 kg is not included in the mass (m) in the numerator. w= √(mgd/I) = √ { (42*10*1)/ [(1/12)(6)(2^2)+42*1] } = √ (420/44) = 3.06- hidemi
- Thread
- Replies: 6
- Forum: Introductory Physics Homework Help
-
H
What is the angular frequency of oscillation?
ω^2 - (ωo)^2 = 2 (-630) (0.32) = -403.2 This is what I have now and I stuck here.- hidemi
- Thread
- Replies: 6
- Forum: Introductory Physics Homework Help
-

Finding Angular Velocity in Rotational Motion Problems
53 rpm equals 5.55 rad/sec multiply 5.55 by 2pi to get angular velocity of 34.8717 Is the answer 34.8717? What should I have done to more accurately solve the problem with a better understanding? What other steps should I take when solving similar problems? and lastly, Is the mass relevant...- momoneedsphysicshelp
- Thread
- Replies: 5
- Forum: Introductory Physics Homework Help
-
F
A Angular power spectrum dependence in redshift z and k
Hi, I wanted to have a precision about a question that has been post on this relation between P(k) and C_l The author writes the ##C_\ell## like this : $$C_\ell(z,z') = \int_0^\infty dkk^2 j_\ell(kz)j_\ell(kz')P(k)$$ I don't undertstand the meaning of ##z## and ##z'## : these are not... -
I
Ballentine Problem 7.1 Orbital Angular Momentum
Find the probability distributions of the orbital angular momentum variables ##L^{2}## and ##L_{z}## for the following orbital state functions: ##\Psi(x) = f(r) sin(\theta) cos(\theta)## ##\Psi(x) = f(r) cos^{2}(\theta)##I am aware that the prob. distribution of an observable is ##|<a_{n} |...- Irishdoug
- Thread
- Replies: 21
- Forum: Advanced Physics Homework Help
-
F
A Calculate variance on the ratio of 2 angular power spectra
In the context of Survey of Dark energy stage IV, I need to evaluate the error on a new observable called "O" which is equal to : \begin{equation} O=\left(\frac{C_{\ell, \mathrm{gal}, \mathrm{sp}}^{\prime}}{C_{\ell, \mathrm{gal}, \mathrm{ph}}^{\prime}}\right)=\left(\frac{b_{s p}}{b_{p... -
E
I Finding the angular momentum of a Kerr black hole
[Mentor Note -- Specialized question moved to the general technical forums] Homework Statement:: To show that ##J = Ma## for the charged Kerr metric [Wald Ch. 11 Pr. 6] Relevant Equations:: \begin{align*} \mathrm{d}s^2 = &- \left( \frac{\Delta - a^2 \sin^2{\theta}}{\Sigma}\right) \mathrm{d}t^2...- etotheipi
- Thread
- Replies: 16
- Forum: Special and General Relativity
-

Engineering Rigid wheel rolling without slipping -- Trying to find angular acceleration
So I tried the problem and it’s different from the solution. I’m confused on why my attempt didn’t work, is it because the wheel is undergoing general planar motion? I tried to just apply Newton’s 2nd law to find the acceleration of the centre and then use that to find angular acceleration. The...- Pipsqueakalchemist
- Thread
- Replies: 15
- Forum: Engineering and Comp Sci Homework Help
-

Engineering Solving for Angular Velocity at Point A - Confused!
So for this question I got the right angular velocity. But I don’t get the same velocity for point A. I don’t understand why it’s cos30, problem asked for V_a when theta = 45 so I used cos45. I have my work below.- Pipsqueakalchemist
- Thread
- Replies: 1
- Forum: Engineering and Comp Sci Homework Help
-

Direction of Angular velocity and Angular momentum?
I am very confused when textbooks say the direction of Angular velocity is perpendicular ot radius and theta for that matter direction is in perpendicular direction. I know this comes from cross product rule but what is the meaning of Angular velocity and Angular momentum directing in upward...- akashpandey
- Thread
- Replies: 9
- Forum: Mechanics
-
S
Shape of graph of torque vs angular displacement of galvanometer
I know the torque will be zero when the deflection is zero and will be maximum when the angular displacement is θ0 but how to determine the exact shape of the graph? Thanks- songoku
- Thread
- Replies: 6
- Forum: Introductory Physics Homework Help
-

I What is the angular velocity of a satellite?
Angular velocity ω is by definition the runned angle dθ per time dt elapsed: ω=dθ/dt. If the time elapsed in the center of the Earth is dt, the dilated time elapsed on satellite is dt′. What is the satellite's angular velocity? Is it dθ/dt or dθ/dt′?- binis
- Thread
- Replies: 132
- Forum: Special and General Relativity
-
H
What is the final angular velocity of the system after the collision?
I calculated as attached and got it right. However, I just wonder why we can't use conservation of energy as the question has already specified 'frictionless', meaning no energy loss and energy distributed to the rotation only.- hidemi
- Thread
- Replies: 4
- Forum: Introductory Physics Homework Help
-
M
The propagator of eigenstates of the Total Angular Momentum
To show that when ##[J^2, H]=0 ## the propagator vanishes unless ##j_1 = j_2## , I did (##\hbar =1##) $$ K(j_1, m_1, j_2 m_2; t) = [jm, e^{-iHt}]= e^{iHt} (e^{iHt} jm e^{-iHt}) - e^{-iHt} jm $$ $$ = e^{iHt}[jm_H - jm] $$ So we have $$ \langle j_1 m_1 | [jm, e^{-iHt} ] | j_2 m_2 \rangle $$ $$ =... -
H
What is the angular momentum of the clay-rod system?
I calculate in this way : Angular Momentum = I W = [ ( 1/12 ML^2 + m(L/2)^2 ] (V/ L/2) = [ 1/12 ML^2 + 1/4 mL^2 ] 2V/L = 2VL/4 [ M/3 + M] but can not find a matching answer. Why?- hidemi
- Thread
- Replies: 12
- Forum: Introductory Physics Homework Help
-

Rotational motion and angular momentum
mball = 2 kg, mputty = 0.05 kg, L = 0.5 m, v = 3m/s a) Moment of inertia : I = (2mball + mputty ). ¼ L^2 = 0.253125 kg.m^2 Linitial = Lfinal => mputty. v. r = I.ω => ω = (4.mputty.v.r) / I = 0.148 rad/s b) K initial = 1/2 m v^2 = 0.225 J K final = 1/2 Iω^2 = 2.85.10^(-3) J => Kfinal /...- hquang001
- Thread
- Replies: 5
- Forum: Introductory Physics Homework Help
-
L
I Angular Momentum Hydrogen Atom Problem: Physically Explained When L=0
In quantum mechanics hydrogen atom problem ##L=\sqrt{l(l+1)}\hbar##. What that means physically when ##L=0##?- LagrangeEuler
- Thread
- Replies: 2
- Forum: Quantum Physics
-

Angular velocity of a weighted rod left free to rotate around a pivot
Hi guys, I don't really know how to solve this problem. The point is finding ##\omega## when ##m_2## passes from ##m_1##'s original position. Ideally, I'm thinking about some conservation of energy/momentum to apply here, but I'm quite confused. Any hint?- greg_rack
- Thread
- Replies: 6
- Forum: Introductory Physics Homework Help
-
M
I Changes in angular momentum for ro-vibrational transitions
Hello! If we have a transition between 2 ro-vibrational levels of the same electronic state of a diatomic molecule the selection rules require for the changes in the rotational quantum number J that ##\Delta J = \pm 1##. Why can't we have ##\Delta J = 0##? The photon carries one unit of angular...- Malamala
- Thread
- Replies: 3
- Forum: Atomic and Condensed Matter
-
L
Circular Motion Questions (energies, forces, angular velocities, etc.)
Question 1: I believe that the ratio would be b. 8:1 because by combining the formula for kinetic energy and momentum the expression Ek=p^2/2m can be obtained. Thus, for a body of mass 2kg with twice the momentum: Ek=2^2/2*2=1 For a body of mass 4kg with half the momentum: Ek=1^2/2*4=1/8...- lpettigrew
- Thread
- Replies: 2
- Forum: Introductory Physics Homework Help
-
S
Angular speed of rod shot by bullet
1) Applying conservation of linear momentum: $$m.u = M.V + m.v$$ where ##V## is final linear speed of the rod $$V=\frac{m.u-m.v}{M}$$2) Applying formula of circular motion: $$V=\omega . r$$ $$\omega = \frac{\left(\frac{mu-mv}{M} \right)}{\frac{1}{2}L-x}$$ Is this correct?And can this be...- songoku
- Thread
-
- Tags
- Angular Angular speed Bullet Rod Speed
- Replies: 11
- Forum: Introductory Physics Homework Help
-

Getting the angular velocity using the angular acceleration graph
The answer here is A What i did is getting the area as follows, 2×4×1/2 +3×-6×1/2 +4×-6 = -29 and then use this Δω=ωf-ωi -29=ωf-5 ωf=24 but there is no such choice.- dahoom102
- Thread
- Replies: 3
- Forum: Introductory Physics Homework Help
-
K
I Electron angular momentum in diatomic molecules
Hello! I just started reading some molecular physics and I am a bit confused about the electron angular momentum in diatomic molecules. Let's say we have just 2 protons and an electron for simplicity and we are in the Born-Oppenheimer approximation, so we assume that the nuclei are fixed in...- kelly0303
- Thread
- Replies: 4
- Forum: Atomic and Condensed Matter
-
A
Exploring Angular Momentum: Examining Earth & Bike Wheels
Take for example earth. Earth has angular momentum about its own axis. However, if we ignore the orbital portion, the angular momentum of the Earth relative to the sun's axis is the same. Another example is the spinning bike wheel/person holding it in a chair. It has angular momentum about its...- alkaspeltzar
- Thread
- Replies: 36
- Forum: Mechanics
-
P
Finding angular speed geometrically
So clearly the easiest way to relate the angular speed to the linear speed would be to start from ##\tan(θ) = x/h## and take a time derivative of both sides. However, it also shouldn't be difficult to find the angular speed geometrically. Using the diagram below one can see that: ##sin(dθ) =...- PhysHobbiest
- Thread
-
- Tags
- Angular Angular speed Speed
- Replies: 4
- Forum: Calculus and Beyond Homework Help
-
O
Angular Width Question in Single Slit Diffraction
i feel like subbing the numbers into the equation isn't enough because of the second minima and maxima thing? not sure what to do... would appreciate any help.- orangegalaxies
- Thread
- Replies: 2
- Forum: Introductory Physics Homework Help
-
C
Solving for $$\omega_2$$ using Conservation of Angular Momentum
Unfortunately, I couldn't arrive to the correct answer ($$=0.28mL^2 \omega^2$$ ) and will be happy to understand what am I doing wrong. **My attempt:** Using $$ E_k = \frac{1}{2} I \omega^2 $$ I obtain that the difference I need to calculate is $$ \frac{1}{2} (2mL^2)(0.8\omega)^2 +...- Combinatorics
- Thread
- Replies: 14
- Forum: Introductory Physics Homework Help
-
A
Spinning Bike Wheel Example, how is angular momentum conserved?
In the classic example of a person holding a spinning bike wheel, as they flip the wheel over, angular momentum is conserved by the person/chair spinning with 2x the angular momentum of the initial wheel. Not questioning that. However, I thought ang momentum is always conserved about a...- alkaspeltzar
- Thread
- Replies: 9
- Forum: Mechanics
-

Real life example of the composition of two SHMs with same angular frequency
Under the topic of simple harmonic motion comes the composition of two SHM's with the same angular frequency, different phase constants, and amplitudes in the same directions and in perpendicular directions. composition of SHM's in same direction: say a particle undergoes two SHM's described by...- Hamiltonian
- Thread
- Replies: 21
- Forum: Mechanics
-
A
Engineering How to find the angular velocity of a rotating drum?
Good day here is the exerciceThe only velocity I do have is the velocity v os the center of pulley 5, I tried to find the center of instantaneous velocity to find the angular velocity of pulley 5 but I couldn't, any hint would be highly appreciated! Best regards!- Amaelle
- Thread
- Replies: 7
- Forum: Engineering and Comp Sci Homework Help
-

Angular momentum of orbit from orbit parameters and mass of sun
L = mvr = mr (dr/dt) = 2m*r*(dr/dt)/2 = 2m*(dA/dt) So, A = (L/2m)T so, ## L = \frac{2 \pi a b m}{T}## Now, ##T^2 = \frac{4 \pi^2}{GM} a^3## So from all these, I get ##L = \sqrt{ \frac{GM m^2 b^2}{a}}## But answer given is ##L = \sqrt{ \frac{2GM m^2 ab}{a+b}}## (This, they have derived from...- Kaguro
- Thread
- Replies: 3
- Forum: Introductory Physics Homework Help
-

Can spin angular momentum get converted to orbital angular momentum?
I know that in QM, there is LS coupling. So the interaction is there. But is such an interaction possible in macroscopic objects like a planet? -

Sum of oscillating frequency and angular velocity
The "egg" initially spun around axis 1 with at ##\omega_s##. After being disturbed, it has started to possesses angular velocities along 2 and 3. The question is to find the rotational speed of ##\vec \omega=\vec\omega_1+\vec\omega_2+\vec\omega_3## to a fixed observer. It is calculated that...- Leo Liu
- Thread
- Replies: 11
- Forum: Introductory Physics Homework Help
-

Why is angular momentum measured at a non inertial CoM conserved?
Question 7.6 Official solution It seems that the solution uses the conservation of angular momentum to solve this question (τ=0). But the problem is that the frame is set on the centre of mass of the guy, which is non inertial. I would like to know why it is correct to do it this way. My...- Leo Liu
- Thread
- Replies: 7
- Forum: Introductory Physics Homework Help
-

Conceptual questions about Angular Momentum Conservation and torque
List of relevant equations: Angular Momentum = L (vector) = r(vector) x p(vector) Angular velocity of rotating object = w(vector), direction found using right hand rule. Torque = T(vector) = dL(vector)/dt I have a few questions about torque and angular momentum direction and...- Ayandas1246
- Thread
- Replies: 2
- Forum: Mechanics
-

Is angular momentum conserved in a non-inertial frame?
Question: If we place the frame of reference on an accelerating point, does the total rotational momentum still remain the same? I attempted to solve this question by manipulating the equations as shown below. $$\text{Define that }\vec r_i=\vec R+\vec r_i'\text{, where r is the position vector... -
J
Angular Momentum: Spinning Mass
First I calculated the momentum of m1. Since m2 was at rest after the collision, all its momentum was transferred, so m1 has a momentum of 158 i hat. L=r x p, so its 916 k hat. This would also be the change in L because it was initially 0 when m1 had no velocity, so I know this is the net...- JoeyBob
- Thread
- Replies: 10
- Forum: Introductory Physics Homework Help
-
J
Angular Momentum Problem: Rotation Rate
First I found the moment of inertia, I=1.8(5.5^2+3.9^2+4.9^2) =125.046 Then I tried to find the rotation rate using the equation L=rotation rate*I rotation rate=3773/125.046=30.173 But the answer is suppose to be 21.263?- JoeyBob
- Thread
- Replies: 5
- Forum: Introductory Physics Homework Help
-
J
Angular Momentum Problem: Torque after fall
I know how to get to the answer but that's what is confusing me. To find final velocity I multiply the acceleration by the time the object fell. Then multiply the velocity by the mass to get momentum. Now the angular momentum is r x p. Since the initial angular momentum was 0, this was also...- JoeyBob
- Thread
- Replies: 4
- Forum: Introductory Physics Homework Help
-
J
Angular Momentum Problem: Determining Angular Acceleration
So I first tried to find L using torque, Torque=d/dt*L, and took the integral of this. Ended up with 23.28484t Now I square the equation L=rotation rate*I to get L^2=rotation acceleration *I^2 Angular acceleration=L^2/I^2 I feel like I am doing something wrong though, this doesn't give the...- JoeyBob
- Thread
- Replies: 13
- Forum: Introductory Physics Homework Help