Calculus Definition and 1000 Threads

Calculus, originally called infinitesimal calculus or "the calculus of infinitesimals", is the mathematical study of continuous change, in the same way that geometry is the study of shape and algebra is the study of generalizations of arithmetic operations.
It has two major branches, differential calculus and integral calculus; the former concerns instantaneous rates of change, and the slopes of curves, while integral calculus concerns accumulation of quantities, and areas under or between curves. These two branches are related to each other by the fundamental theorem of calculus, and they make use of the fundamental notions of convergence of infinite sequences and infinite series to a well-defined limit.Infinitesimal calculus was developed independently in the late 17th century by Isaac Newton and Gottfried Wilhelm Leibniz. Today, calculus has widespread uses in science, engineering, and economics.In mathematics education, calculus denotes courses of elementary mathematical analysis, which are mainly devoted to the study of functions and limits. The word calculus (plural calculi) is a Latin word, meaning originally "small pebble" (this meaning is kept in medicine – see Calculus (medicine)). Because such pebbles were used for counting (or measuring) a distance travelled by transportation devices in use in ancient Rome, the meaning of the word has evolved and today usually means a method of computation. It is therefore used for naming specific methods of calculation and related theories, such as propositional calculus, Ricci calculus, calculus of variations, lambda calculus, and process calculus.

View More On Wikipedia.org
Recent contents Top users
  1. S

    Calculus Calculus textbooks with good sections on integration

    Hi I'm having troubles with integration specially by substitution, I'm going to read a calculus textbook and i need recommendations of books with a good treatment on the different techniques of integration. I'd like a book with good exercises for self study and a exposure to integration of...
  2. Leo Liu

    Computing the polar moment of inertia (calculus)

    Question: Diagram: So the common approach to this problem is using polar coordinates. The definition of infinitesimal rotational inertia at O is ##dI_O=r^2\sigma\, dA##. Therefore the r. inertia of the triangle is $$I_O=\int_{0}^{\pi/3}\int_{0}^{\sec\theta}r^2r\,drd\theta$$ whose value is...
  3. G

    I Skipping elementary calculus and starting at analysis?

    I was walking around with my head in the clouds and suddenly I wondered if a smart person, say, a philosopher, could start at the full monster of real analysis instead of elementary calculus. Would there be any hope for this unfortunate soul? What are your opinions and why? Or if you feel this...
  4. S

    "Introduction to Calculus and Analysis" by Courant & Fritz

    Hi, I'm reading the volume 1 of "Introduction to calculus and analysis" by Courant and Fritz but the problems are hard for me, i understand what he say but i can't solve many problems of the chapter one. It's normal or should i try with other book?
  5. Adgorn

    Calculus Looking for a rigorous multivariable calculus book

    Hello everyone. I'm about to take Calc 3 next semester and am looking for a rigorous book to work with on multivariable calculus. I've gone through Spivak's "Calculus" from cover to cover and am hoping to find something with the same degree of rigor, if possible, and preferably with a solution...
  6. F

    How to approach vector calculus identities?

    Majoring in electrical engineering imply studying Griffiths book on electrodynamics, so I have begun reading its first chapter, which is a review of vector calculus. A list of vector calculus identities is given, and I would like to derive each one, with one of them being ##\nabla \cdot (A...
  7. S

    Equations for a mass falling to Earth from a distance

    I have a question : If we consider the change in g due to distance from the Earth core; then y=distance from earth’s core t=time G=gravitation constant M=Earth’s mass k=GM $$y^2(t)=\frac{k}{y(t)^2}$$ If we consider air resistive force as proportional to speed squared, then: m=falling object...
  8. C

    Can you use Taylor Series with mathematical objects other than points?

    I was recently studying the pressure gradient force, and I found it interesting (though this may be incorrect) that you can use a Taylor expansion to pretend that the value of the internal pressure of the fluid does not matter at all, because the internal pressure forces that are a part of the...
  9. yucheng

    Prove the lower bound for a sequence (Buck, Advanced Calculus)

    Clearly, ##x_{n+1}>x_n \because x_n + \sqrt{x_n} > x_n## $$ \begin{align*} x_{n+1} &= x_n+ \sqrt{x_n} \\ &= x_1 + \sqrt{x_1} + \sqrt{x_2} + \cdots \sqrt{x_n} \\ &>n+1 \end{align*} $$ ##\because \sqrt{x_n}>\sqrt{x_1}=1## In fact, $$x_{n+1} > 1+ \sqrt{1} + \sqrt{2}+ \sqrt{3} + \cdots \sqrt{n}$$...
  10. K

    Calculus Best Calculus of variations (Sturm Liouville Theory) textbook?

    Hi, I have a course on calculus of variations and Sturm Liouville theory and was wondering if anyone had any good textbook suggestions? If they had questions and solutions it would be a bonus! I have put all the subtopics of the course below. Calculus of variations Variation subject to...
  11. T

    A Exploring Tensor Calculus: A Brief Introduction

    Hello.Questions: How tensor operations are done?Like addition, contraction,tensor product, lowering and raising indices. Why do we need lower and upper indices if we want and not only lower? Is a tensor a multilinear mapping?Or a generalisation of a vector and a matrix? Could a tensor be...
  12. R

    B Is Multivariable Calculus as Fun as Single-Variable Calculus?

    I've been studying calculus A and B on and off over the last ten years, and I'm starting to learn calculus again for fun as soon as I can get my hands on a textbook. I was wondering if multivariable calc is as fun as A and B have been so far.
  13. SirMadame

    I Self-Study General Relativity: After Multivariate Calc in HS

    I just finished multivariate calculus (without any linear algebra experience yet) and I am seeking out a path to understanding General Relativity. I am wondering what are the mathematical fields after multivariate calculus that I need to master before beginning to understand GR, and what...
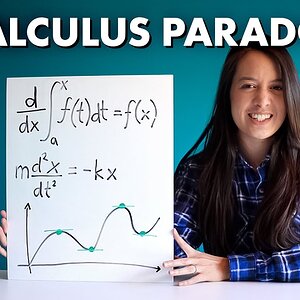
  14. Three paradoxes explained with Calculus.

    Three paradoxes explained with Calculus.

    Up and Atom gives a look at some Calculus tools which a layman can follow.
    • scottdave
    • Media item
    • calculus limits zeno
    • Comments: 0
    • Category: Calculus
  15. avata4

    How to Solve Calculus Problems with Recurrence: A Step-by-Step Explanation

    i think solution with récurrence for n=1 then 1=2¨¨^0(2x0 +1) true suppose that n=2¨^p(2q+1) is true shows that n+1=2^p( 2q +1)? n+1=2¨^p(2q+1) +1 ⇒ ??
  16. T

    I Euler, Calculus of Variations and Mast on a ship

    From Wikipedia: "In 1727, [Euler] first entered the Paris Academy Prize Problem competition; the problem that year was to find the best way to place the masts on a ship." Does anyone know how he did this? Is there an on-line paper? (But what that is accessible with today's knowledge). And by...
  17. cwill53

    Integrating Mass of a Hollow Sphere: Multivariable Calculus Explained

    I know some multivariable calculus, I just want someone to walk me through the integration deriving the mass element dM and the integration of thin rings composing the hollow sphere. It would also be nice if you could show me doing it one way using the solid angle and one way without using the...
  18. barryj

    Please explain this calculus solution

    If f'(x) were a simpler function like f'(x) = cos(x) I would say f(x) = sin(x) + C and then evaluate C by knowing that 2 = sin(1) + C and then C would equal 2-sin(1) the f(x) = sin(x) + 2 - sin(1), f(0) = sin(0) + 2 - sin(1) = 0 + 2 -.841 = 1.58 However the more complicated problem has f'(x) -...
  19. WMDhamnekar

    MHB Does the Cosine Rule Apply to Vector Addition in 3-D?

    Hi, In $\mathbb{R^3} || v-w ||^2=||v||^2 + ||w||^2 - 2||v||\cdot ||w||\cos{\theta}$ But can we say $||v+w||^2=||v||^2 +||w||^2 + 2||v|| \cdot||w|| \cos{\theta}$ where v and w are any two vectors in $\mathbb{R}^3$
  20. mcastillo356

    Calculus Finding an Alternative to "Calculus" by Robert A. Adams

    Hi PF, Can you tell me about an alternative, substitute for "Calculus", written by Robert A. Adams, from University of British Columbia?. It's good, but I need more bibliography; I find this one too implicit: suggested but not communicated directly. I am now asking doubts to a lot of forums each...
  21. L

    Calculus and Kinematic equations--- seeing the logic

    Details of Question: ds/dt= v which becomes ds=v dt, where s=displacement, t =time, and v=velocity Then we can integrate both sides of this equation, and do a little algebra, and turn the above equation into: s − s0 = v0t + ½at2 My main question is about the integration of...
  22. A

    MHB Calculus 3 Help: Iterated, Double, Triple Integrals & More

    Kindly help me with: Iterated integrals Q1, 2,3 Double integrals in polar coordinates Q1, 2,3 Triple integrals Q1, 2,3 Triple integrals in cylindrical coordinates Q1, 2,3 Triple integrals in spherical coordinates Q1, 2,3 Change of variables Q7,8,9 Green's theorem Q1,2 Surface integrals...
  23. TheGreatDeadOne

    Calculating Gradient of 1/|r-r'|: Tips & Results

    Doing R=|r-r'|, i get the expected result: \nabla \frac{1}{|r-r'|} = -\frac{1}{R^2}\hat r=-\frac{(r-r')}{|r-r'|^3} But doing it this way seems extremely wrong, as I seem to be disregarding the module. So I tried to do it by the chain rule, and I got: \nabla...
  24. J

    What are the key topics in Advanced Calculus and Algebraic Geometry?

    Hello, I am a very experienced Mathematician with a BSc Honours degree in Mathematics and one year MSc studies in Operational Research in Sussex and London Universities respectively. I am interested in Advanced Calculus, Algebras, Positivity in Algebraic Geometry, The standard Model, and many...
  25. Hamiltonian

    B Basic doubts in vector and multi variable calculus

    If say we have a scalar function ##T(x,y,z)## (say the temperature in a room). then the rate at which T changes in a particular direction is given by the above equation) say You move in the ##Y##direction then ##T## does not change in the ##x## and ##z## directions hence ##dT = \frac{\partial...
  26. A

    MHB Master Calculus Questions with Expert Tips | Boost Your Grades

  27. Baums Mizushala

    Point on a graph nearest to the origin

    The Attempt at a Solution I know the answer is supposed to be ##(-1,0)##. However when I differentiate the above expression I get. $$ 2x+{\frac 5 2} $$ Then the shortest distance would be when the expression equates to 0. $$ 2x+{\frac 5 2}=0 $$ I should be getting ##x=-1## but solving for ##x##...
  28. ElieQuebec10

    I Calculus: What is the derivative of ##\phi## at a point?

  29. karush

    MHB 3.3.2 AP Calculus Exam interval from f'(x)

    screen shot to avoid typos OK the key said it was D I surfed for about half hour trying to find a solution to this but $f'(0)$ doesn't equal any of these numbers $e^0=\pm 1$ from the $e^{(x^2-1)^2}$ kinda ?
  30. karush

    MHB S8.7.2.1 AP calculus Exam (typo problem)

    ok I thot this was just observation to get b. but maybe not I saw some rather hefty substations to get different answers
  31. karush

    MHB What Is the Value of the Integral $\int_0^1 xe^x \, dx$?

    $\tiny{4.2.5}$ $\displaystyle\int^1_0{xe^x\ dx}$ is equal to $A.\ \ {1}\quad B. \ \ {-1}\quad C. \ \ {2-e}\quad D.\ \ {\dfrac{e^2}{2}}\quad E.\ \ {e-1}$ ok I think this is ok possible typos but curious if this could be solve not using IBP since the only variable is x
  32. Quarkman1

    Greetings from a 'late learner' and physics fan

    Hello! I am a 'mature' learner and am fascinated by all kinds of physics and math ideas. Learning is the key to enjoying science and keeping an open mind. I must admit, I am not very sharp on my physics skills and my calculus is pretty rusty now (I don't work in the science field, per se) so I...
  33. T

    Evaluate the Taylor series and find the error at a given point

    I have the following function $$f^{(0)}\left(x\right)=f\left(x\right)=e^{x}$$ And want to approximate it using Taylor at the point ##\frac{1}{\sqrt e} ## I also want to decide (without calculator)whether the error in the approximation is smaller than ##\frac{1}{25} ## The Taylor polynomial is...
  34. J

    A How Do You Solve This Alternating Series Involving Logarithms?

    Hi! Some time ago I came across a series and never solved it, I tried to give a new go because I was genuinely curious how to tackle it, which I thought would work, because it looks innocent, but there is something about the beast making it hard to approach for me. So need some help! Maybe this...
  35. JorgeM

    A How do I express an equation in Polar coordinates as a Cartesian one.

    I got a polar function. $$ \psi = P(\theta )R(r) $$ When I calculate the Laplacian: $$ \ \vec \nabla^2 \psi = P(\theta)R^{\prime\prime}(r) + \frac{P(\theta)R^{\prime}(r)}{r} + \frac{R(r)P^{\prime\prime}(\theta)}{r^{2}} $$ Now I need to convert this one into cartesian coordinates and then...
  36. EchoRush

    Help with deriving the formula for kinetic energy (using calculus)

    Hello, I am learning how to use calculus to derive the formula for kinetic energy now, I understandthe majority of the steps in how to do this, however, there is one step where I get totally lost, I will post a picture of the steps and I will circle the part where I get lost. If you see the...
  37. jaychay

    MHB Calculus airplane related rates problem ( cosine rule)

    A student has test his airplane and he is far from the airplane for 5 meter.He start to test his airplane by letting his airplane to move 60 degree from the horizontal plane with constant velocity for 120 meter per minute.Find the rate of distance between the student and the plane when the plane...
  38. jaychay

    Related rates calculus problem about a water tank

    Summary:: Consider the rectangular water tank, at the base the length is the same for 200 cm. There are 100 holes for water to come out which each hole have the same flow rate. Find the amount of water that come out in each hole by using differential when we know that there is an error in the...
  39. jaychay

    MHB Related rates calculus problem about water tank

    Consider the rectangular water tank, at the base the length is the same for 200 cm. There are 100 holes for water to come out which each hole have the same flow rate. Find the amount of water that come out in each hole by using differential when we know that there is an error in the measurement...
  40. K

    Nabla operations, vector calculus problem

    Here is how my teacher solved this: I understand what the nabla operator does, ##∇\cdot\vec v## means that I am supposed to calculate ##\sum_{n=1}^3\frac {d\vec v} {dx_n}## where ##x_n## are cylindrical coordinates and ##\vec e_3 = \vec e_z##. I understand why ##∇\cdot\vec v = 0##, I would get...
  41. Bright Liu

    How do I derive this vector calculus identity?

    ##(\nabla\times\vec B) \times \vec B=\nabla \cdot (\vec B\vec B -\frac 1 2B^2\mathcal I)-(\nabla \cdot \vec B)\vec B## ##\mathcal I## is the unit tensor
  42. R

    I Deriving Lorentz Transformations Using Calculus

    We take an arbitrary spacetime point ##(x,t)## in any observer's reference frame ##A##. Let ##(x(v),t(v))## be the co-ordinates of this same event as seen from a frame ##B## moving at a velocity ##v## wrt ##A##. As ##v## varies, the set of points ##(x(v),t(v))## constitute some curve ##C##. So...
  43. rxh140630

    Calculus Apostol's vol 1 calculus not as good as Stewart's calculus?

    Hello, all around the web and even on this website, I've been told countless times that Apostol/Spivak's calculus books are superior to Stewarts. Having personally read about a forth of Apostol's book, and having read half or more of Stewarts, I notice Stewart has better explanations, and better...
  44. karush

    MHB 1.6.1 AP Calculus Exam Limits with L'H

    $\displaystyle\lim_{x \to 0}\dfrac{1-\cos^2(2x)}{(2x)^2}=$ by quick observation it is seen that this will go to $\dfrac{0}{0)}$ so L'H rule becomes the tool to use but first steps were illusive the calculator returned 1 for the Limit
  45. S

    Finding the Determinant to find out if the matrix is invertible

    question: My first attempt: my second attempt: So I am getting 0 (the right answer) for the first method and 40 for the second method. According to the theorem, shouldn't the determinant of the matrix remain the same when the multiple of one row is added to another row? Can anyone explain...
  46. S

    MHB Single Variable Calculus Summary Rulesheet

    Students see my 20+ page calculus bundle on limits, derivatives and integrals and their applications. The summary notes are cleanly written, have background math grid paper, and summarize all major concepts, formulas, and procedures from calculus books. Please tell me what you think and if this...
  47. karush

    MHB Q1 Can you pass this 3 question AP calculus Quiz in 10 minutes

    1. $f(x)=(2x+1)^3$ and let g be the inverse function of f. Given that$f(0)=1$ what is the value of $g'(1)$? A $-\dfrac{2}{27}$ B $\dfrac{1}{54}$ C $\dfrac{1}{27}$ D $\dfrac{1}{6}$ E 6 2. given that $\left[f(x)=x-2,\quad g(x)=\dfrac{x}{x^2+1}\right]$ find $f(g(-2))$...
  48. S

    A Fractional Calculus - Variable order derivatives and integrals

    Does anyone know any good research on this topic? I'm basically looking for information on what would be solving integral and differential equations in which the unknown you need to solve for is the level of a integral or derivative in the equation. For example F'1/2(u)+F'x(u)=F'1/3(u) where the...
  49. Sabertooth

    "Astronomical Calculus" Spaceship Dilation problem

    Hi everyone. I have provided myself a problem that I insist on solving, however, I want to do it "the right way" where I can put every parameter into a calculator and get an answer quickly. I pondered doing it manually and figured that it could be done to a reasonable precision in an hour or...
  50. karush

    MHB 2.4.3 AP Calculus Exam Integration limits

    by observation I choose (c) since the limit values may not be =
Back
Top