Classical Definition and 1000 Threads
-
K
A Non holonomic constraints in classical mechanics textbook
I want to learn about the non holonomic case in lagrangian and Hamiltonian mechanics. I've seen that many people say that Goldstein 3rd ed is wrong there. Where should I go to learn it. My mathematics level is at the level Goldstein uses. Please help- Kashmir
- Thread
- Classical Classical mechanics Constraints Hamiltonian formalism Mechanics Textbook
- Replies: 2
- Forum: Classical Physics
-
V
Books that teach classical mechanics through a discourse method
Books that teaches classical mechanics through a discourse method ie asking interesting questions and answering them maybe a similar one to Understanding Basic Chemistry Through Problem Solving: The Learner's Approach Book by Jeanne Tan and Kim Seng Chan. Not exactly asking numerical questions...- Viishnuuu
- Thread
- Books Classical Classical mechanics Mechanics Method
- Replies: 10
- Forum: Science and Math Textbooks
-

I Classical Aberration Formula: Understanding & Application
I believe I have understood the formula of aberration of light ##\tan \theta' = \dfrac{\sin \theta}{\beta + \cos \theta} \sqrt{1-\beta^{2}} ## but I wonder if the non-relativistic formula ## \tan \theta' = \dfrac{\sin \theta}{\beta + \cos \theta} ## has a physical relevance. Does this...- Kairos
- Thread
- Aberration Classical Formula
- Replies: 7
- Forum: Special and General Relativity
-

Work & Energy (Question on Classical Mechanics/Slope based Problems)
I used the Change in Kinetic Energy and equated that with the Work Done. The "Work Done" part comprises of two different functions- one is work done by Gravitational Force while the other is the work done by frictional force (or the brakes). /Delta KE (magnitude wise)= 0.5*1350* (20^2)=270,000...- warhammer
- Thread
- Classical Classical mechanics Energy Introductory physics Work
- Replies: 7
- Forum: Introductory Physics Homework Help
-
L
I Help with Goldstein Classical Mechanics Exercise 1.7
I'm trying to solve the Goldstein classical mechanics exercises 1.7. The problem is to prove: $$\frac{\partial \dot T}{\partial \dot q} - 2\frac{\partial T}{\partial q} = Q$$ Below is my progress, and I got stuck at one of the step. Now since we have langrange equation: $$\frac{d}{dt}... -
A
B Is Relativity the Evolution of Classical Physics?
Can someone please tell me what is Classical Physics? Does Kinematics in One Dimension such as in Physics textbook is considered a "Classical Physics"?- askor
- Thread
- Classical Classical physics Physics
- Replies: 9
- Forum: Classical Physics
-

B Need a bet settled about classical momentum
Consider a car slamming into an unyielding wall at 60 mph. Objects in the car will be slammed against the dashboard with a certain amount of force. Now, instead of slamming into a stationary wall, you slam into another car coming towards you at 60 mph. Relative speed, 120MPH. QUESTION: Will...- Paige_Turner
- Thread
- Classical Momentum
- Replies: 7
- Forum: Classical Physics
-

A Proof of Classical Fluctuation-Dissipation Theorem
Sorry if there's latex errors. My internet connection is so bad I can't preview. Here's the wikipedia proof I'm referring to. I'm fine with the steps up to $$W(x,0) = W_0 (x) [1 + \beta f_0 (x(0) - \langle x \rangle_0) ]$$ where ##W(x,t)## is the probability density of finding the system at...- Twigg
- Thread
- Classical Proof Theorem
- Replies: 1
- Forum: Classical Physics
-
G
B What Math do I need to learn best for Classical and Quantum Physics?
I am a 14 year old who is very interested in Physics, especially astrophysics, but I don’t know exactly which math I should study in order to learn more in depth of the field. What is the best thing to learn?- Godzilla-3301
- Thread
- Classical Physics Quantum Quantum physics
- Replies: 5
- Forum: General Math
-

Understanding the Differences Between Bose and Classical Gases
In classical statistics, we derived the partition function of an ideal gas. Then using the MB statistics and the definition of the partition function, we wrote: $$S = k_BlnZ_N + \beta k_B E$$, where ##Z_N## is the N-particle partition function. Here ##Z_N=Z^N## This led to the Gibb's paradox...- Kaguro
- Thread
- Classical Entropy Gas
- Replies: 2
- Forum: Introductory Physics Homework Help
-
Building a motorcycle, need classical mechanics help
Hi! I am an engineering graduate that took my bachelor's degree in Mechanical Engineering much too long ago, but I have forgotten a lot of the classical mechanics/mechanics of materials theory that I had learned many years ago. I am building a motorcycle right now, and I want to calculate the...- Feroyn
- Thread
- Building Classical Classical mechanics Mechanics Motorcycle
- Replies: 4
- Forum: Mechanical Engineering
-
H
I Time Reversal Breaking in Classical Systems
I am looking for an example of a classical system without time reversal symmetry. I would appreciate any help.- hokhani
- Thread
- Classical Systems Time Time reversal
- Replies: 5
- Forum: Classical Physics
-
J
A 1D transverse-field Ising model - classical vs quantum differences?
The 1D transverse field Ising model $$ H(\sigma)=-J\sum_{i\in \mathbb{Z}} \sigma^x_i \sigma^x_{i+1} -h \sum_{i \in \mathbb{Z}} \sigma^z_i$$ is usually solved in quantum way, but we can also solve it classically - e.g. parametrize angles of spins ##\sigma^x_i = \cos(\alpha_i)...- Jarek 31
- Thread
- 1d Classical Ising model Model Quantum Spin
- Replies: 1
- Forum: Atomic and Condensed Matter
-
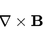
Density of states of one three-dimensional classical harmonic oscillator
ia- anaisabel
- Thread
- Classical Density Density of states Harmonic Harmonic oscillator Oscillator States Statisical mechanics
- Replies: 11
- Forum: Advanced Physics Homework Help
-

Speed of a hanging rope sliding on a nail (using energy conservation)
I solved this problem easily using Newton's second law, but I had problems trying to use mechanical energy conservation to solve it. How I solved using Newton's second law: ##\text{(part of the rope that is on the left)}\, m_1=x\rho g,\, \text{(part of the rope that is on the right)}\...- TheGreatDeadOne
- Thread
- Classical Classical mechanics Conservation Conservation of energy Energy Energy conservation Rope Sliding Speed
- Replies: 6
- Forum: Introductory Physics Homework Help
-
I
Classical Reading Goldstein's Classical Mechanics as an Undergraduate
We were prescribed Goldstein, Taylor and Marion/Thornton for our first course in analytical mechanics, and I'm about to finish up the course but I feel like I have not gotten a good physical, intuitive grasps of the concepts, so I've been trying to read the texts a bit more. Taylor and...- IAmLoco
- Thread
- Classical Classical mechanics Mechanics Reading Undergraduate
- Replies: 33
- Forum: Science and Math Textbooks
-

I Is the classical relation between energy and momentum valid in QM?
Here we are talking about non-relativistic quantum physics. So we all know kinetic energy T = E - V = \frac{1}{2}mv^2 in classical physics. Here V is the potential energy of the particle and E is the total energy. Now what I am seeing is that this exact same relation is being used in quantum...- shankk
- Thread
- Classical Energy Free particle Momentum Momentum and energy Qm Relation Stationary states
- Replies: 4
- Forum: Quantum Physics
-
V
I Classical Hayden-Preskill Randomizer
I am reading this paper: https://arxiv.org/abs/0708.4025. In section 2, they describe a classical black hole as a classical randomizer. The black hole initially is a ##n-k## bit string, and Alice sends a ##k##-bit string into the black hole. A permutation of all ##2^n## possible strings is the...- vancouver_water
- Thread
- Classical
- Replies: 1
- Forum: Quantum Physics
-

A Something about configuration manifolds in classical mechanics
I think it could be interesting. Consider a mechanical system A circle of mass M can rotate about the vertical axis. The angle of rotation is coordinated by the angle ##\psi##. A bead of mass m>0 can slide along this circle. The position of the bead relative the circle is given by the angle...- wrobel
- Thread
- Classical Classical mechanics Configuration Manifolds Mechanics
- Replies: 2
- Forum: Differential Geometry
-

Electrical Permittivity of Classical Vacuum - Physical?
The Vacuum Permittivity of the classical vacuum seems to be for just conversion of units in Coulomb's Law, like Coulomb's Constant in disguise. Does the Electrical Permittivity of the Vacuum in classical electromagnetism have any real physical significance other than in the above context...- LarryS
- Thread
- Classical Electrical Permittivity Physical Vacuum
- Replies: 16
- Forum: Electromagnetism
-
W
Does any classical mechanics textbook solve Kepler's Problem?
I have several* classical physics and mechanics texts, and none solve the Kepler problem (as far as I can tell), succinctly, solving the Kepler equation, M = E - e*sin(E), for E given M and e, or more generally determining the equations of motion for an orbiting object. In fact none even...- Will Flannery
- Thread
- Classical Classical mechanics Mechanics Textbook
- Replies: 22
- Forum: STEM Educators and Teaching
-
B
Photoelectric Effect Classical Breakdown
So I'm kind of confused about how to interpret the question and the idea of there being a small enough ##\tau## for the classical picture to break down. I started with the max KE eqn: ##KE_{max} = E_{incoming} - \phi## I suppose ##E_{incoming}## is the power (W) times time and that is also...- baseballfan_ny
- Thread
- Classical Photoelectric Photoelectric effect Planck
- Replies: 8
- Forum: Introductory Physics Homework Help
-
E
B What are your favourite classical physics puzzles?
^ :wink: The title is pretty self-explanatory, wondered if people would like to share some fun problems that you can snuggle up to on a cold Winter's evening? Any difficulty level, but bonus points for problems which yield to elegant and/or creative solutions! Here's an example:- etotheipi
- Thread
- Classical Classical physics Physics
- Replies: 32
- Forum: Classical Physics
-
J
Energy paradox in classical electrodynamics?
Consider two massive charged objects at rest with a large horizontal distance ##d## between them (object ##1##: mass ##m_1##, charge ##q_1## and object ##2##: mass ##m_2##, charge ##q_2##). I apply a constant vertical force ##\vec{f_1}## upwards to object ##1## so that it gains an acceleration...- jcap
- Thread
- Classical Classical electrodynamics Electrodynamics Energy Paradox
- Replies: 19
- Forum: Electromagnetism
-
E
I Classical equivalent of scalar free field in QFT
Hi there, In QFT, a free scalar field can be represented by the lagrangian density $$\mathcal{L} = \frac{1}{2}\left(\partial\phi\right)^2 - \frac{1}{2}m^2\phi^2$$ I would like to find a classical system that has the same lagrangian. If we consider the transversal motion of an elastic string...- eoghan
- Thread
- Classical Equivalent Field Qft Scalar Scalar field
- Replies: 5
- Forum: Quantum Physics
-
E
Classical explanation for microwave door shielding
What is the classical explanation for why the microwaves won't pass through the microwave door? -

Classical Some books on introductory classical physics
I need some suggestions on classical physics. You can help me with some high school papers or books to get started with.Thanks!- sahilmm15
- Thread
- Books Classical Classical physics Introductory Physics
- Replies: 7
- Forum: Science and Math Textbooks
-
A
How to implement a Fredkin gate in a classical network?
Hi, I'm wondering about the Fredkin gate (controlled swap) gate, which is defined by the truth table on p54 here. I'm trying to implement it in a simple feedback network that takes the form of what can be input into this software: integratedinformationtheory.org/calculate. Any pointers would be...- Agrippa
- Thread
- Classical Gate Network
- Replies: 7
- Forum: Programming and Computer Science
-

Classical Konopinski Classical Descriptions of Motion (1969)
Does anyone have any experience with this book? It looks interesting. See attached TOC- Frabjous
- Thread
- Classical Motion
- Replies: 1
- Forum: Science and Math Textbooks
-

I How Can LASER Be Both a Quantum and Classical Light Source?
My question is the physics behind the LASER such as stimulated emission can be only explained by quantum mechanics only. We can represent LASER as coherent state in quantum mechanics only. Then how can we say LASER can be thought of a classical light source?- Muthumanimaran
- Thread
- Atomic Classical Laser Mechanics Physics Quantum Quantum mechanics
- Replies: 4
- Forum: Atomic and Condensed Matter
-
S
Classical Recommend books about classical mechanics please
Hi! i need some textbooks recommendations to learn by my self about classical mechanics in a undegraduate level. I don´¨¨t know what kind of math is required, i have knowledge about calculus by my high school classes and i learned more with the book "Calculus" by Gilbert Strang. I wait for your...- Santiago24
- Thread
- Books Classical Classical mechanics Mechanics
- Replies: 11
- Forum: Science and Math Textbooks
-
H
A Ads/cft correspondence and quantum / classical correspondance
Hi Pf I do not know if it is only an analogy but i consider these two correspondences: I) the transformation of a quantum spin into a statistical problem by a Wick rotation. we start with a qubit evolving in time between 0 and t from S0 to St. we use a exp(iHt) operator to describe its...- Heidi
- Thread
- Ads/cft Classical Quantum
- Replies: 18
- Forum: Beyond the Standard Models
-
A
Does a statistical mechanics of classical fields exist?
The usual presentation of classical statistical mechanics are based on the Liouville equation and phase space distribution. This, in turn, is based on the Hamiltonian mechanics of a system of point particles. Real undulatory systems, specially non-linear ones, have to be complex to study... -
B
I Interpretation of quantum theories through the interpretation of classical ones
Perhaps the reason is that the principle of least action is elevated to a divine principle that does not require materialistic interpretation. If so, then the solution to the "secrets" of quantum theories lies in the solution of the materialistic essence of the physical magnitude of action.- bayakiv
- Thread
- Classical Interpretation Quantum Theories
- Replies: 19
- Forum: Quantum Interpretations and Foundations
-
J
I Born rule in classical Ising model: Feynman -> Boltzmann ensemble?
Quantum mechanics is often said to be equivalent with Feynman path ensemble, which "after Wick rotation" becomes Boltzmann path ensemble, also called euclidean path integrals (popular for numerical calculations), or random walk/diffusion MERW (maximal entropy random walk). But Boltzmann path...- Jarek 31
- Thread
- Boltzmann Born rule Classical Ensemble Feynman Ising model Model
- Replies: 2
- Forum: Quantum Physics
-
E
Classical Prerequisites for Arnold's Methods of Classical Mechanics
I've finished with Gregory's classical mechanics and was looking for something a bit more challenging. I thought Arnold's methods of classical mechanics look pretty interesting, but it's definitely more mathematically complex than anything I would have done before, especially the bits about...- etotheipi
- Thread
- Classical Classical mechanics Mechanics Prerequisites
- Replies: 11
- Forum: Science and Math Textbooks
-

MHB Is the Given Answer for the Classical Mechanics Problem on Earth Correct?
- WMDhamnekar
- Thread
- Classical Classical mechanics Mechanics
- Replies: 3
- Forum: General Math
-

I Quantum vs Classical multiphoton interference on double slit
For a setup analogous to double-slit experiment, do I understand it correctly that if we have two photons, one going only through slit A and another going only through slit B simultaneously (or vice versa as they have a symmetrical wave function), then there will be no interference pattern...- MichPod
- Thread
- Classical Double slit Interference Quantum Slit
- Replies: 17
- Forum: Quantum Physics
-

Deriving Statistical Behavior of Particles via Classical Mechanics
Hello, using computation simulation, can the statistical behavior of many particles be derived through deterministic classical mechanics? -
S
Classical mechanics -- Equations for simulating the motion of a body
Hello forum, i want to make a samulation of a body. The body will be moved horisontal on y,x axis. I want on my simulation the body to change direction many times(for example i want to go for 10sec right and then left end right...). My question is does i need more than one differential equation... -

Jackson Classical Electrodynamics: page 35 expansion of charge
Could anyone explain how did Jackson obtain the Taylor distribution of charge distribution at the end of section 1.7 (version 3)?- qnach
- Thread
- Charge Classical Classical electrodynamics Electrodynamics Expansion Jackson Poisson equation
- Replies: 5
- Forum: Electromagnetism
-

Music Longest piece of classical music changes chords today
the d# a# e chord held since 2013 will change today to g# e. The next chord change is February 5, 2022. The piece will end in 620 years on Sep 5, 2640 https://www.aslsp.org/de/ https://en.wikipedia.org/wiki/As_Slow_as_Possible- BWV
- Thread
- Classical Music
- Replies: 21
- Forum: Art, Music, History, and Linguistics
-

Are there articles on Classical computation of the Photoelectric Effect?
photoelectric effect is now explained in quantum idea. Why the classical model fails? Are there articles computing it in detail using classical model?- thaiqi
- Thread
- Articles Classical Computation Photoelectric Photoelectric effect
- Replies: 21
- Forum: Electromagnetism
-

Can We Cancel the Derivative of dt in These Equations?
problem in this book : classical mechanics goldstein Why can we cancel the derivative of dt from these equations? e.g. ##\frac{d(x)}{dt} + \frac{b sin\theta}{2} \frac{d(\theta)}{dt} = asin\theta \frac{d(\phi)}{dt}## ## x +\frac{b \theta sin\theta}{2} = a \phi sin\theta ## because I think... -

Classical Canonical Partition Function in Two Dimensions
For a single particle, $$Z=\frac{1}{h^2}\int_{-\infty}^{\infty} e^{-\beta \frac{P^2}{2m}}d^2p \int e^{-U(r)}drd\theta= \frac{1}{h^2}(\frac{2\pi m}{\beta}) 2\pi [\int_{0}^{r_0}e^{U_0}dr+\int_{r_0}^{R}dr]$$ $$ =\frac{1}{h^2}(\frac{2\pi m}{\beta}) 2\pi [e^{U_0}(r_0)+(R-r_0)]=\frac{\pi...- Diracobama2181
- Thread
- Classical Dimensions Function Partition Partition function Two dimensions
- Replies: 4
- Forum: Advanced Physics Homework Help
-
C
Classical Predictions of Scattered Radiation
I am currently reading the third edition of Modern Physics by Serway/Moses/Moyer and this quote, in reference to the Compton Effect, seems to conflict with various other online sources I have checked. I understand that the classical description does not give accurate predictions of what actually...- CWK
- Thread
- Classical Compton effect Radiation
- Replies: 5
- Forum: Thermodynamics
-

I Quantum spaces without classical counterparts?
Hi to all members! From few days that I am thinking on this question: there are finite-dimensional spaces that are quantum spaces (models for QM) but without the classical counterpart in classical mechanics ? For example I know that entanglement have not a ''clearly'' classical counterpart ...- Ssnow
- Thread
- Classical Quantum
- Replies: 5
- Forum: Quantum Physics
-

Classical Classical electrodynamics for high-energy physicists
I've just found a recent very interesting and very modern textbook on classical electrodynamics. It starts with special relativity (rather than electrostatics) and contains a lot of high-energy topics, including renormalization (within classical realm), massive vector fields, gravitational...- Demystifier
- Thread
- Classical Classical electrodynamics Electrodynamics Physicists
- Replies: 2
- Forum: Science and Math Textbooks
-

David Morin classical mechanics Problem 2.6: Disk held up by a massless string
The first part is easy, we have 2T= Mg T= 0.5 Mg Now for the second part where I'm having trouble understanding Morin's solution: I take the normal force on a small circle arc to be N, we know that the y component of the normal force must be balance with Mg for the whole disk, therefore Ny =...- John100861
- Thread
- Classical Classical mechanics Disk Massless Mechanics Morin Statics String Tension
- Replies: 5
- Forum: Introductory Physics Homework Help
-
S
Classical Supplement to Classical Mechanics by Goldstein
Are there any lecture notes that closely follow Classical Mechanics by Goldstein? I am asking this since I am seeing some comments in this forum that it contains some conceptual errors, e.g. nonholonomic constraints. If there is a book that "closely" follows Goldstein, it will be good too.- shinobi20
- Thread
- Classical Classical mechanics Goldstein Mechanics
- Replies: 16
- Forum: Science and Math Textbooks